F. Sadlo,
G. K. Karch,
T. Ertl:
In Topological Methods in Data Analysis and Visualization III, Springer International Publishing,
pp. 217–231,
2014.

|
|
|
Abstract
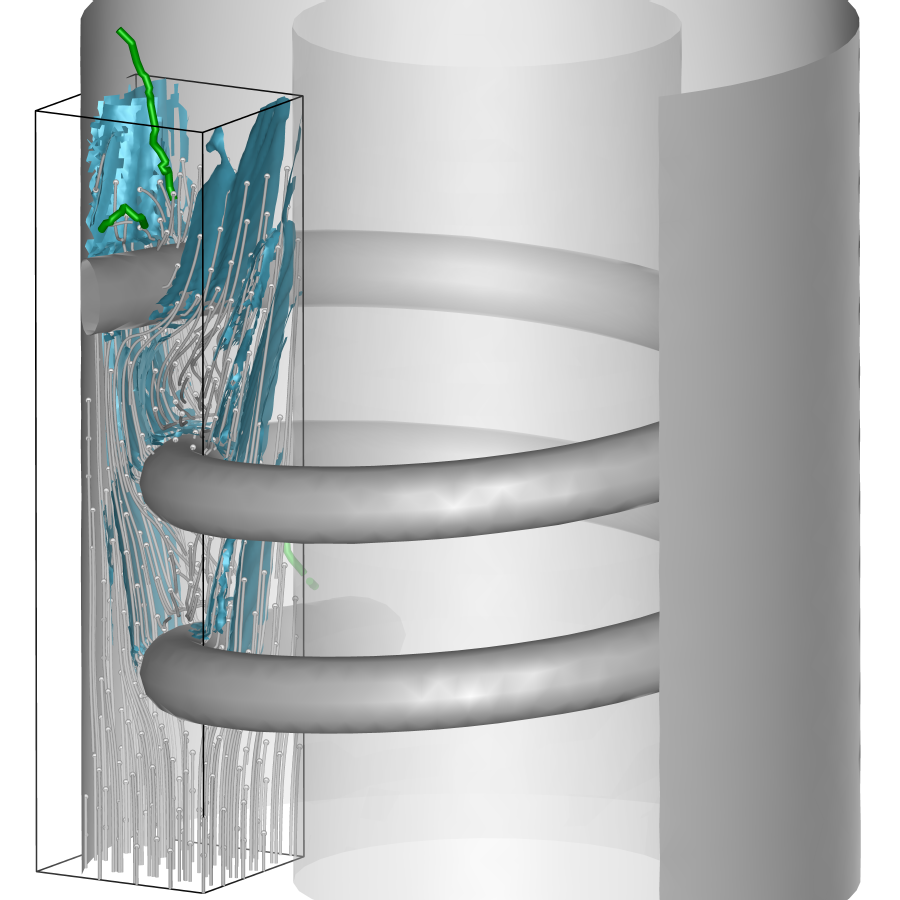
Concepts from vector field topology have been successfully applied to a wide range of phenomena so far—typically to problems involving the transport of a quantity, such as in flow fields, or to problems concerning the instantaneous structure, such as in the case of electric fields. However, transport of quantities in time-dependent flows has so far been topologically analyzed in terms of advection only, restricting the approach to quantities that are solely governed by advection. Nevertheless, the majority of quantities transported in flows undergoes simultaneous diffusion, leading to advection-diffusion problems. By extending topology-based concepts with diffusion, we provide an approach for visualizing the mechanisms in advection-diffusion flow. This helps answering many typical questions in science and engineering that have so far not been amenable to adequate visualization. We exemplify the utility of our technique by applying it to simulation data of advection-diffusion problems from different fields.
|
Available Files
[BibTeX]
[DOI]
[PDF]
|
|


